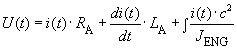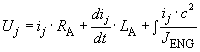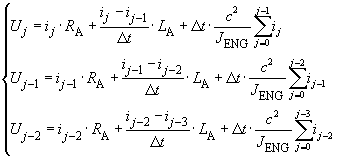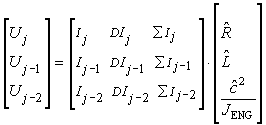Identification of the parameters of the second order dynamic object by difference schemes

E.V. Bolovin, A.S. Glazyrin.

Department of Electric Drives and Equipment, National Research Tomsk Polytechnic University, Tomsk, Russia

Abstract

In this paper a method for determining the parameters of the second-order dynamic object is developed based difference schemes.

Key words: second-order dynamic object, identification of the parameters, difference schemes.

Introduction

In modern production necessary using drives. Different motors are used in drives, both in structure and purpose, and by age category. Effective control of these motors is possible if you know the values of their parameters. But, often determine the parameters is impossible or very difficult. It is connected with the new imported motors, where only the specified power and speed, and the aged motors which have served ten years in which the passport data, were either lost or took the motor is not one-time repairs. Accordingly, the acute question of identification of their parameters. The aim of this paper is selection the optimal method for identifying parameters of objects in the electric control.

Identification of the parameters of the second order dynamic object

The second-order dynamic object - the DC motor with independent excitation was considered

The system of differential equations (SDE) describing the motor was written

(1)

where c- coefficient of coupling between the armature EMF and speed of DC-motor
- voltage applied to the motor armature, - the armature current, - rotational speed of the armature of DC-motor, - the load torque applied to the motor shaft, - the equivalent moment of inertia.

In SDE the first equation - the equation of electric equilibrium, composed of the second law of Kirchhoff, the second - the equation of motion of the engine.

For determining the parameters of the engine (R_A, L_A, J_ENG) convert (1) so as to get rid of speed and its derivative. This can be done as follows.

Express the second derivative of the velocity equation

The first equation was differentiated and substitute the derivative

Get rid of the derivative on the left side of the equation

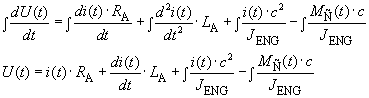
Since M_C = 0

(2)

Make a discretization of equation (2), the values of the armature voltage and current DC-motor will be determined at time

(3)

where - the sampling interval or time step, j - number of time step.

(4)

There is an integral in the resulting equation (4), but it’s unacceptable for further solutions. To get rid of it by one of the methods of sampling, which is the immediate replacement of all the variables of discrete values, where the derivative is replaced by the difference between the left of the first order, and the integral - the amount calculated by the method of rectangles. Thus, we obtain the j-th step, given the - the step time.

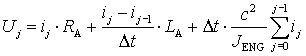
Since the three unknown parameters, namely the R_A, L_A, J_eng, needs a system of three equations.

	(5)
(5) was transformed in the form of vectors:	(6)

Here

(6) was solved by the inverse matrix

where,

Conclusion

The procedure of compilation the difference equations for identification the parameters of the dynamic object of the second order is presented. This procedure can be applied to DSP-controlled motor drives.