Physics / 6.radiophysics

Bubis E.L.

IAP RAS Nizhny Novgorod, Russia

Study of the phase contrast technique with photothermal filters

The phase contrast method proposed by F.Zernike in 1934 is widely used for visualization of optical small-scale inhomogeneities of a medium (both inherent and induced) in various fields of physics and engineering from optical microscopy, measurement of weak absorption of light by a medium, to plasma physics [1-5]. The phase contrast technique based on nonlinear Zernike cells (filters) started to develop recently [6-15]. As distinct from the schemes employing linear filters (in the simplest case, Zernike plates responsible for a selective phase shift of quarter a wavelength between zero and higher space frequencies participating in the object imaging formation), nonlinear Zernike filters are optical schemes requiring less adjustment (all-optical, self-adaptive phase contrast imaging technique), with the necessary phase shift provided by choosing an appropriate intensity of light entering the nonlinear medium. The use of nonlinear Zernike cells for analyzing a light wave phase in adaptive optical systems was proposed in [2]. The present paper deals with results of the experimental study of a phase-contrast scheme with a photothermal cell, in which phase mismatch is due to a thermal nonlinearity mechanism in an absorbing medium. Numerical calculations concerning some problems of image quality in such systems, taking into account nonlocal response of a nonlinear medium are given in [12]. Thermal processes in pure liquids in the field of long laser pulses have been previously considered by the author (see, e.g., [15]).

A one-lens scheme of image formation shown in fig. 1 and analogous to the scheme with a photothermal cell located in the Fourier plane of the system [6-8] was employed in the experiment.

Phase

object

The objects were illuminated by a Gaussian beam of a single-mode linearly polarized He – Ne laser of the power P≤ 6mW and the wavelength λ = 0,63μm. The radiation passed through an object was focused by a lens (F=6 cm, D=3.5 cm) to the middle of a cell filled with ethyl alcohol or acetone and an absorber. The value ≈ 0.2 – 0.6 was chosen experimentally. Here is the absorption coefficient of the medium and is its length. A cell with an absorbing medium =1-100 mm was used. Images of examined transparent objects visualized on a screen located at a distance of 10 m from the lens were photographed with a digital camera. Figures 2 show visualized images of a cross of two light guides (100) placed in a quartz cell filled with vaseline oil at the radiation a) and b) ; in the case b) one distinctly observes the effect of image inversion with some distortions caused by beam self-action in the medium, с)linear dark-field method.

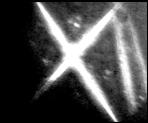
All the results are obtained at a power that does not lead to a marked self-action of the beam in the absence of a phase object. At the incident power Р≥ 5 mW a typical aberration picture of thermal defocusing was seen in the far zone.

Therefore, basic properties of a phase contrast scheme with photothermal Zernike cell are studied. The required level of scheme operation corresponds to the initial stage of developing thermal self-action of an illuminating laser beam, which is an important and most low-threshold nonlinear-optical phenomenon for continuous and quasi-continuous laser radiation.

References

[1] Francon M. Le Microscope a Contraste de Phase et Le Microscope Interferentiel. Paris: Editiondu center national de la recherence scientifique. 1954.

[2]Vorontsov M.A., Koryabin M.A., Shmalhauzen V.I. Controlled optical systems. M.: Nauka. 1988.

[3] H. Weisen.// Rev.Sci.Instrum.,1988, 59(8), pp. 1544 – 1549.

[4] Roland C.Anderson and Steven Lewis //Appl.Opt,1985,Vol.24,No.22, 3687.

[5] Babin A.A., Bubis E.L., Lozhkarev V.V.et al // Quantum Electronics. 1998. V. 28, №8. Р. 738–740.

[6] Tchernega N.V., et al. // Quantum Electronics , 1989, №12, с. 2530 -2538.

[7] Iturbe-Castillo M.D.et al.// Opt.Eng. 2001, Vol 40. N 11. P. 2367 – 2368. ; Treviño-Palacios C.G. et al // Appl.Opt., 2003, Vol.42, No.25, pp.5091 – 5095.

[8] Bubis E.L. // Preprint of the Institute of Applied physics of RAS. N. Novgorod. 2006. № 698.

[9] Yelleswarapu C.S.et al //Applied Physics Letters. 2006. V. 89. P. 211116-1.

[10] Bubis E.L., Matveev A.Z. // Tech.Phys.Lett. 2007, Vol.33, No.6, p.454.

[11] Bubis E.L.,et al // Proc. SPIE, Vol. 6729, 2007. p.52.

[12] Bubis E.L., Matveev A.Z. // Proc.SPIE, 2007, Vol. 6729, p.82.

[13] Bubis E.L // Tech.Phys.Lett. 2008, Vol.34, No.6,pp.510 – 511.

[14] Bubis E.L // Instruments and Experimental Techniques, 2009, Vol. 52, No. 1, pp. 108–109

[15] Bubis E.L.,et al // Optics and Spectroscopy, 1988, Том 65, №6, с. 1281 -1286.